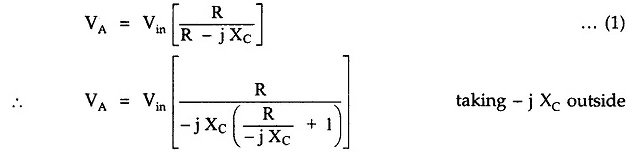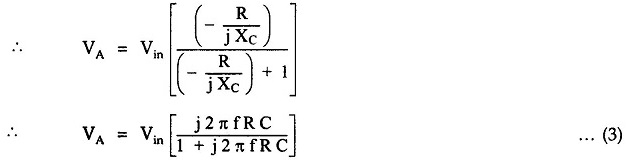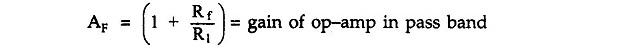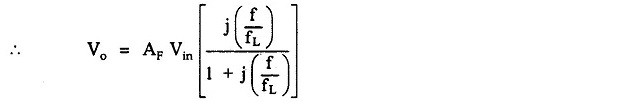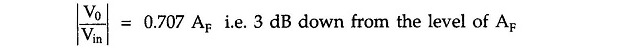First Order High Pass Butterworth Filter:

As mentioned earlier, a high pass filter is a circuit that attenuates all the signals below a specified cut off frequency denoted as fL. Thus, a high pass filter performs the opposite function to that of low pass filter. Hence, the First Order High Pass Butterworth Filter circuit can be obtained by interchanging frequency determining resistances and capacitors in low pass filter circuit.

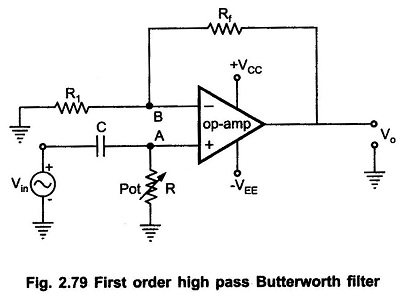
First Order High Pass Butterworth Filter

The first order high pass filter can be obtained by interchanging the elements R and C in a first order low pass filter circuit. The Fig. 2.79 shows the first order high pass Butterworth filter.

It can be observed that as compared to first order low pass filter, the positions of R and C are changed in the high pass circuit shown in Fig. 2.79.

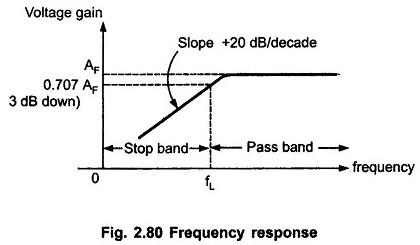
The frequency at which the gain is 0.707 times the gain of filter in pass band is called as low cut off frequency and denoted as fL. So, all the frequencies greater than fL are allowed to pass but the maximum frequency which is allowed to pass is determined by the closed loop bandwidth of the op-amp used.

Analysis of the Filter Circuit:

The impedance of the capacitor is

First Order High Pass Butterworth Filter

where f is the input i.e. operating frequency.

By the voltage divider rule, the potential of the non inverting terminal of the op-amp is

As

Substituting in the above expression of VA,.

This can be represented as

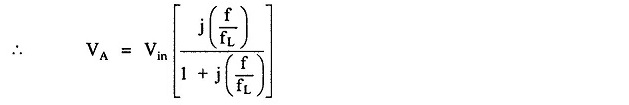
First Order High Pass Butterworth Filter

where

Now, for the op-amp in non-inverting configuration,

First Order High Pass Butterworth Filter

where

and

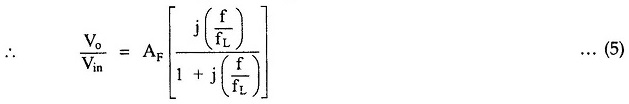
First Order High Pass Butterworth Filter

This is the required expression for the transfer function of the filter. For the frequency response, we require the magnitude of the transfer function which is given by,

First Order High Pass Butterworth Filter

The equation (6) describes  the behavior of the high pass filter.

1.At low frequencies, i.e. f < f′L

First Order High Pass Butterworth Filter

2) At f < f′L

First Order High Pass Butterworth Filter

3) At f > fL, i.e. high frequencies, 1 can be neglected as compared to (f/fL) from denominator.

First Order High Pass Butterworth Filter

Thus, the circuit acts as high pass filter with a passband gain as AF. For the frequencies, f < fL, the gain increases till f = fL at a rate of + 20 dB/decade. Hence, the slope of the frequency response in stop band is + 20 dB/decade for first order high pass filter. The frequency response is shown in the Fig. 2.80.

Frequency Response of First Order High Pass Butterworth Filter

Note : As high pass filter is basically a low pass filter circuit with positions of R and C interchanged, the design steps and the frequency scaling method discussed earlier for low pass filter is equally applicable to the first order high pass Butterworth filter.

Related posts:

  1. Second Order High Pass Butterworth Filter
  2. First Order High Pass Butterworth Filter Derivation
  3. Second Order High Pass Butterworth Filter Derivation
  4. First Order Low Pass Butterworth Filter
  5. Second Order Low Pass Butterworth Filter
  6. Second Order Low Pass Butterworth Filter Derivation
  7. Frequency Response for Low Pass Filter and High Pass Filter
  8. High Pass Filter
  9. m Derived High Pass Filter
  10. Higher Order Filter Design
  11. Low Pass Filter Circuits
  12. Band Pass Filter Circuit Diagram
  13. All Pass Filter Design
  14. Low Pass Filter
  15. Band Pass Filter
  16. m Derived Low Pass Filter
  17. m Derived Band Pass Filter
  18. Normalized Low Pass Filter Characteristics
  19. High Pass RC Circuit Diagram, Derivation and Application
  20. RC High Pass Circuit as Differentiator