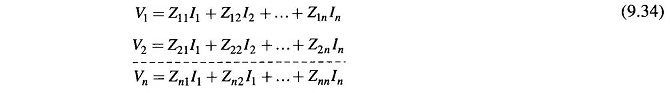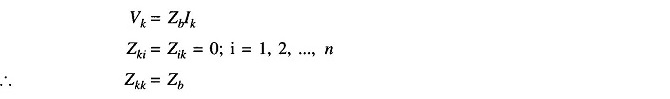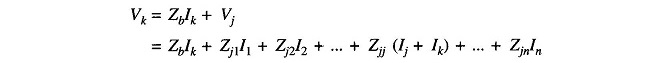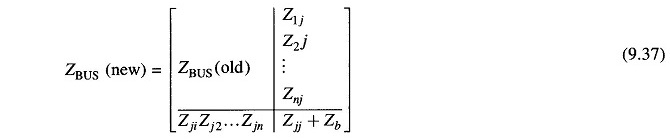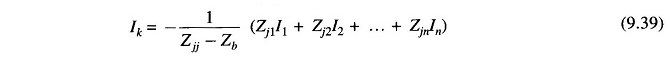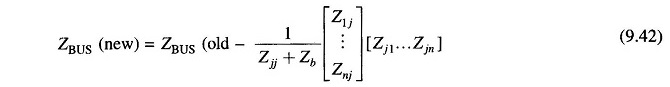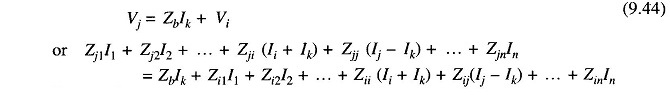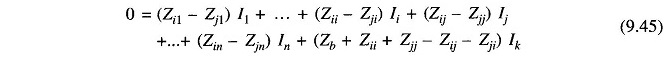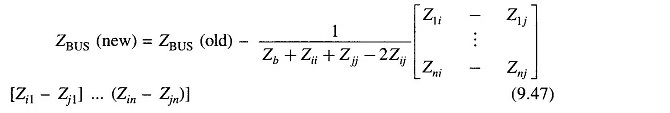Z_BUS Formulation:

Z_BUS Formulation is given by

By Inventing Y_BUS

The sparsity of Y_BUS may be retained by using an efficient inversion technique and nodal impedance matrix can then be calculated directly from the factorized admittance matrix.

Current Injection Method:

Equation (9.33) can be written in the expanded form

It immediately follows from Eq. (9.34) that

Also Z_ij = Z_ji; (Z_BUS Formulation is a symmetrical matrix).

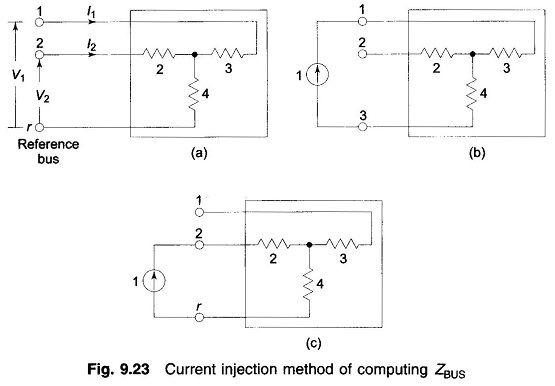
As per Eq. (9.35) if a unit current is injected at bus (node) j, while the other buses are kept open circuited, the bus voltages yield the values of the jth column of Z_BUS. However, no organized computerizable techniques are possible for finding the bus voltages. The technique had utility in AC Network Analyzers where the bus voltages could be read by a voltmeter.

Z_BUS Building Algorithm:

It is a step-by-step programmable technique which proceeds branch by branch. It has the advantage that any modification of the network does not require complete rebuilding of Z_BUS Formulation.

Consider that Z_BUS Formulation has been formulated up to a certain stage and another branch is now added. Then

Upon adding a new branch, one of the following situations is presented.

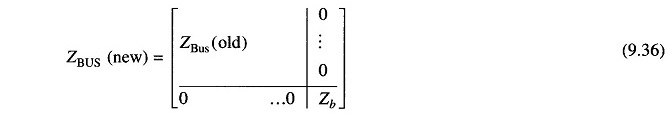
1. Z_b is added from a new bus to the reference bus (i.e. a new branch is added and the dimension of Z_BUS goes up by one). This is type-I modification.
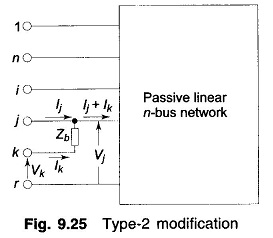
2. Z_b is added from a new bus to an old bus (i.e., a new branch is added and the dimension of Z_BUS goes up by one). This is type-2 modification.
3. Z_b connects an old bus to the reference branch (i.e., a new loop is formed but the dimension of Z_BUS does not change). This is type-3 modification.
4. Z_b connects two old buses (i.e., new loop is formed but the dimension of Z_BUS does not change). This is type-4 modification.
5. Z_b connects two new buses (Z_BUS remains unaffected in this case). This situation can be avoided by suitable numbering of buses and from now on wards will be ignored.

Notation: i, j—old buses; r—reference bus; k—new bus.

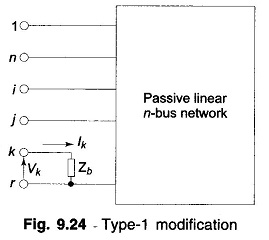
Type-1 Modification:

Figure 9.24 shows a passive (linear) n-bus network in which branch with impedance Z_b is added to the new bus k and the reference bus r. Now

Hence

Type-2 Modification:

Z_b is added from new bus k to the old bus j as in Fig. 9.25. It follows from this figure that

Rearranging,

Consequently

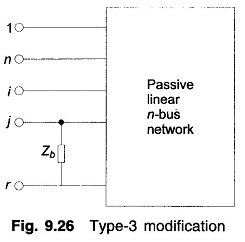
Type–3 Modification:

Z_b connects an old bus (j) to the reference bus (r) as in Fig. 9.26. This case follows from Fig. 9.25 by connecting bus k to the reference bus r, i.e. by setting V_k = 0.

Thus

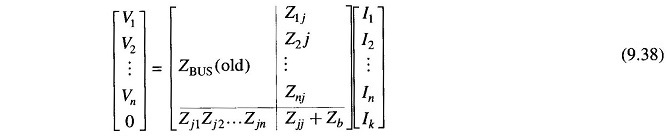
Eliminate I_k in the set of equations contained in the matrix operation (9.38),

or

Now

Substituting Eq. (9.40) in Eq. (9.39)

Equation (9.37) can be written in matrix form as

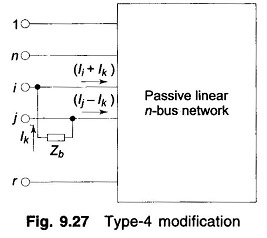
Type-4 Modification:

Z_b connects two old buses as in Fig. 9.27. Equations can be written as follows for all the network buses.

Similar equations follow for other buses.

The voltages of the buses i and j are, however, constrained by the equation (Fig. 9.27)

Rearranging

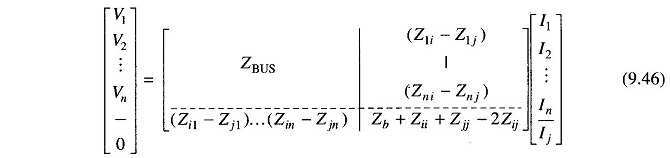
Collecting equations similar to Eq. (9.43) and Eq. (9.45) we can write

Eliminating I_k in Eq. (9.46) on lines similar to what was done in Type-2 modification, it follows that

With the use of four relationships Eqs (9.36), (9.37), (9.42) and (9.47) bus impedance matrix can be built by a step-by-step procedure

When the network undergoes changes, the modification procedures can be employed to revise the bus impedance matrix of the network. The opening of a line (Z_ij) is equivalent to adding a branch in parallel to it with impedance — Z_ij.