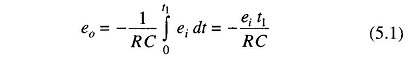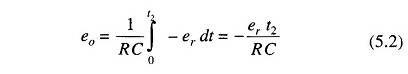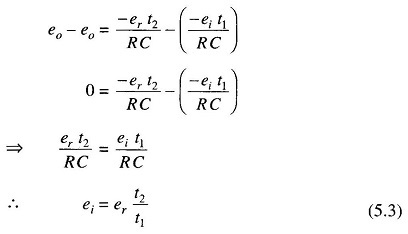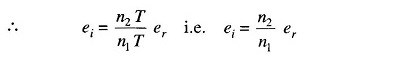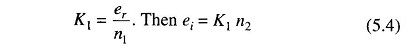Dual Slope Integrating Type DVM (Voltage to Time Conversion):

Dual Slope Integrating Type DVM – In ramp techniques, superimposed noise can cause large errors. In the dual ramp technique, noise is averaged out by the positive and negative ramps using the process of integration.

Principle of Dual Slope Type DVM:

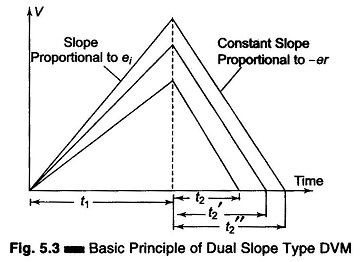
As illustrated in Fig. 5.3, the input voltage ’e_i’ is integrated, with the slope of the integrator output proportional to the test input voltage. After a fixed time,

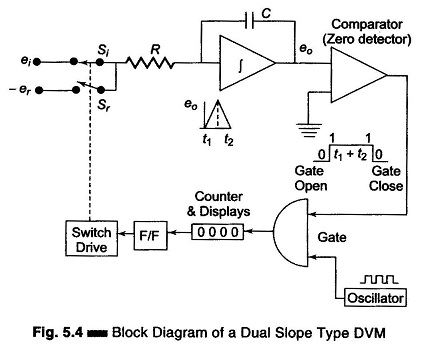
equal to t₁, the input voltage is disconnected and the integrator input is con­nected to a negative voltage – e_r The integrator output will have a negative slope which is constant and proportional to the magnitude of the input voltage. The block diagram is given in Fig. 5.4.

At the start a pulse resets the counter and the F/F output to logic level ‘0’. S_i is closed and S_r is open. The capacitor begins to charge. As soon as the integrator output exceeds zero, the comparator output voltage changes state, which opens the gate so that the oscillator clock pulses are fed to the counter. (When the ramp voltage starts, the comparator goes to state 1, the gate opens and clock pulse drives the counter.) When the counter reaches maximum count,

i.e. the counter is made to run for a time ‘t₁‘ in this case 9999, on the next clock pulse all digits go to 0000 and the counter activates the F/F to logic level ‘1’. This activates the switch drive, e_i is disconnected and –e_r is connected to the integrator. The integrator output will have a negative slope which is constant, i.e. integrator output now decreases linearly to 0 volts. Comparator output state changes again and locks the gate. The discharge time t₂ is now proportional to the input voltage. The counter indicates the count during time t₂. When the negative slope of the integrator reaches zero, the comparator switches to state 0 and the gate closes, i.e. the capacitor C is now discharged with a constant slope. As soon as the comparator input (zero detector) finds that e_o, is zero, the counter is stopped. The pulses counted by the counter thus have a direct relation with the input voltage.

During charging

During discharging

Subtracting Eqs 5.2 from 5.1 we have

If the oscillator period equals T and the digital counter indicates n₁ and n₂ counts respectively,

Now, n₁ and e_r are constants.

Let

From Eq. 5.3 it is evident that the accuracy of the measured voltage is inde­pendent of the integrator time constant. The times t₁ and t₂ are measured by the count of the clock given by the numbers n₁ and n₂ respectively. The clock oscil­lator period equals T and if n₁ and e_r are constants, then Eq. 5.4 indicates that the accuracy of the method is also independent of the oscillator frequency.

The dual slope technique has excellent noise rejection because noise and superimposed ac are averaged out in the process of integration. The speed and accuracy are readily varied according to specific requirements; also an accuracy of ± 0.05% in 100 ms is available.