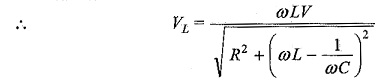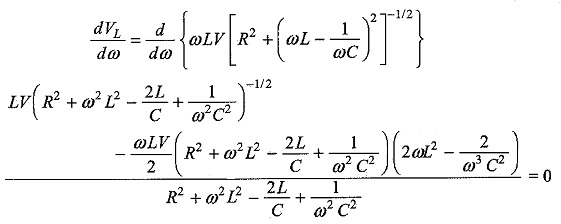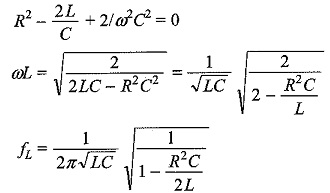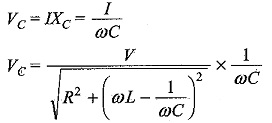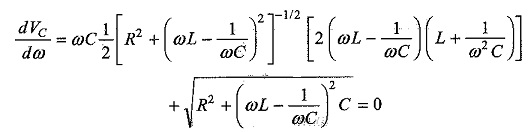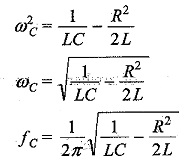Voltage and Current in Series Resonant Circuit:

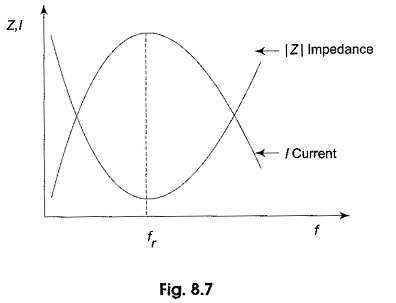
The variation of impedance and current with frequency of Voltage and Current in Series Resonant Circuit is shown in Fig. 8.7.

At resonant frequency, the capacitive reactance is equal to inductive reactance, and hence the impedance is minimum. Because of minimum impedance, maximum current flows through the circuit. The current variation with frequency is plotted.

The voltage drop across resistance, inductance and capacitance also varies with frequency. At f = 0, the capacitor acts as an open circuit and blocks current. The complete source voltage appears across the capacitor. As the frequency increases, X_C decreases and X_L increases, causing total reactance X_C — X_L to decrease. As a result, the impedance decreases and the current increases. As the current increases, V_R also increases, and both V_C and V_L increase.

When the frequency reaches its resonant value f_r, the impedance is equal to R, and hence, the current reaches its maximum value, and V_R is at its maximum value.

As the frequency is increased above resonance, X_L continues to increase and X_C continues to decrease, causing the total reactance, X_L—X_C to increase. As a result there is an increase in impedance and a decrease in current. As the current decreases, V_R also decreases, and both V_C and V_L decrease. As the frequency becomes very high, the current approaches zero, both V_R and V_C approach zero, and V_L approaches V_S.

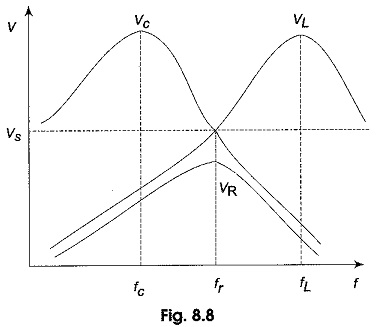
The response of different voltages with frequency is shown in Fig. 8.8.

The drop across the resistance reaches its maximum when f = f_r. The maximum voltage across the capacitor occurs at f = f_c. Similarly, the maximum voltage across the inductor occurs at f = f_L.

The voltage drop across the inductor is

where

To obtain the condition for maximum voltage across the inductor, we have to take the derivative of the above equation with respect to frequency, and make it equal to zero.

If we solve for ω, we obtain the value of ω when V_L is maximum.

From this

Similarly, the voltage across the capacitor is

To get maximum value dV_c/dω = 0

If we solve for ω, we obtain the value of ω when V_C is maximum.

From this

The maximum voltage across the capacitor occurs below the resonant frequency; and the maximum voltage across the inductor occurs above the resonant frequency.