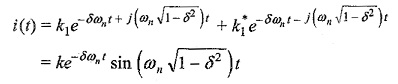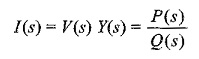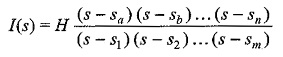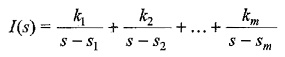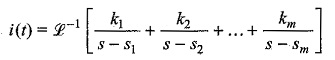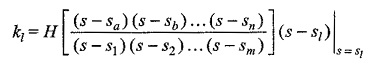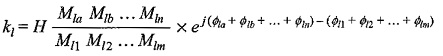Poles and Zeros of Time Domain Response:

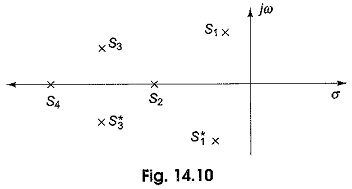
For the given network function, a pole zero plot can be drawn which gives useful information regarding the critical frequencies. The Poles and Zeros of Time Domain Response can also be obtained from pole zero plot of a network function. Consider an array of poles shown in Fig. 14.10.

In Fig. 14.10 s₁ and s₃ are complex conjugate poles, whereas s₂ and s₄ are real poles. If the poles are real, the quadratic function is

where δ is the damping ratio and ω_n is the undamped natural frequency.

The roots of the equation are

For these poles, the time domain response is given by

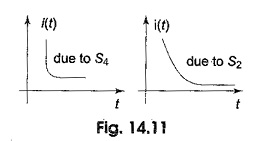
The response due to pole s₄ dies faster compared to that of s₂ as shown in Fig. 14.11.

s₁ and s₃ constitute complex conjugate poles. If the poles are complex conjugate, then the quadratic function is

The roots are

For these poles, the time domain response is given by

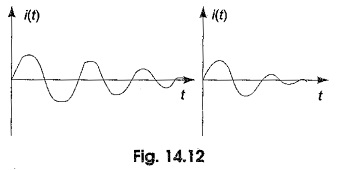
From the above equation, we can conclude that the response for the conjugate poles is damped sinusoid. Similarly, s₃, s₃^* are also a complex conjugate pair. Here the response due to s₃ dies down faster than that due to s₁ as shown in Fig. 14.12.

Consider a network having transfer admittance Y(s). If the input voltage V(s) is applied to the network, the corresponding current is given by

This may be taken as

where H is the scale factor.

By taking the partial fractions, we get

The time domain response can be obtained by taking the inverse transform

Any of the above coefficients can be obtained by using Heavisides method. To find the coefficient k_l

Here s_l, s_m, s_n are all complex numbers, the difference of (s_l – s_n) is also a complex number.

Hence

Similarly, all coefficients k₁,k₂,….k_m may be obtained, which constitute the

magnitude and phase angle.

The residues may also be obtained by Poles and Zeros of Time Domain Response in the following way.

1. Obtain the pole zero plot for the given network function.
2. Measure the distances M_la, M_lb,….,M_ln of a given pole from each of the other zeros.
3. Measure the distances M_l1, M_l2,….,M_lm of a given pole from each of the other poles.
4. Measure the angle Φ_la, Φ_lb,….,Φ_ln of the line joining that pole to each of the other zeros.
5. Measure the angle Φ_l1, Φ_l2,….,Φ_lm of the line joining that pole to each of the
   other poles.
6. Substitute these values in required residue equation.

Amplitude and Phase Response from Pole Zero Plot:

The steady state response can be obtained from the pole zero plot, and it is given by

where M(ω) is the amplitude

Φ(ω) is the phase

These amplitude and phase responses are useful in the design and analysis of network functions. For different values of ω, corresponding values of M(ω) and Φ(ω) can be obtained and these are plotted to get amplitude and phase response of the given network.