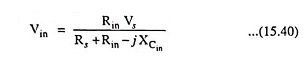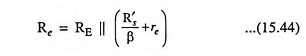Low Frequency Response of BJT Amplifier:

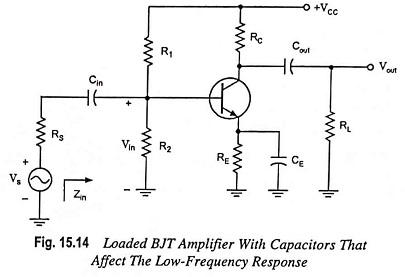
For analysis, we will consider the loaded voltage-divider BJT bias configuration. But the results can be applied to any configuration. For the network shown in Fig. 15.14 the capacitors C_in, C_out and C_E will determine the low frequency response of BJT Amplifier.

Now we will examine the impact of each independently.

Effect of C_in on Frequency Response of Amplifier:

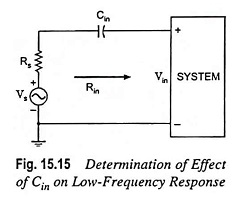
Since C_in is normally con­nected between the applied source and the active device, the general form of the R-C combination is established by the network shown in Fig. 15.15.

The total resistance is now (R_s + R_in) and the cutoff frequency is

At mid or high frequencies, the reactance of the capacitor C_in will be considerably small to allow a short-circuit approximation for the element. The relation between V_in and V_s is given as

At f_Li, input voltage V_in will be 0.707 times the value determined by above Eq. (15.38), assuming that C_in is the only capacitive element that controls the Low Frequency Response of BJT Amplifier.

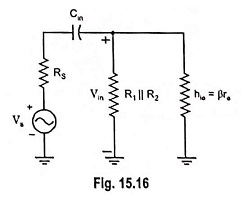
For the network given in Fig. 15.14, in analysis of the effects of C_in, we must assume that the capacitors C_E and C_out are performing their de-signed function or the analysis becomes too unwieldy, that is, that the magnitudes of the reactances of C_out and C_E allow using a short-cir­cuit equivalent as compared to the magnitude of the other series impedances. Using this hypothesis, the ac equivalent network for the input section of the circuit shown in Fig. 15.14 will become as shown in Fig. 15.16.

The value of R_in is given by the equation

The voltage V_in applied to the input of device can be determined by using voltage-divider rule and is given as

Thus with the decrease in frequency, the reactance of the capacitor C_in increases, some of the signal or source voltage is lost across the input capacitor C_in and the voltage V_in applied to the input of the device is reduced resulting in decrease in output voltage and hence the gain.

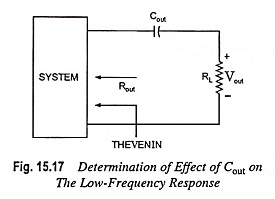
Effect of C_out on Frequency Response of Amplifier:

Since the output capacitor is normally connected between the output of the active device and the load, the R-C configuration determining the lower cutoff frequency due to C_out will appear as given in Fig. 15.17. From Fig. 15.17, the total series resistance is now R_out + R_L and the cutoff frequency due to C_out is given by equation

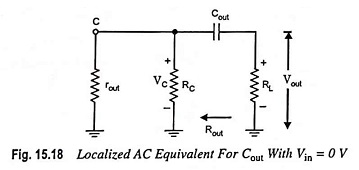
Ignoring the effects of C_in and C_E, the output voltage will be 70.7% of its midband value at f_Lo. For the network shown in Fig. 15.14, the ac equivalent network for the output section with V_in = 0, will appear as shown in Fig. 15.18.

The value of R_out is given by the equation

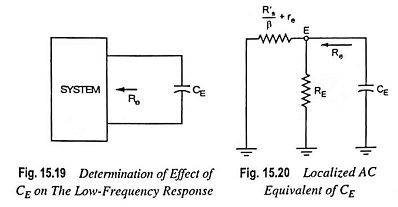
Effect of C_E on Frequency Response of Amplifier:

For determination of frequency f_Le, the network “seen” by C_E must be determined as illustrated in Fig. 15.19.

Once the level of R_e is determined, the cutoff frequency due to C_E can be computed from the relation

For the network shown in Fig. 15.14, the ac equivalent as ‘seen’ by C_E will be as given in Fig. 15.20.

The value of R_e is given by the equation

where R′_s = R_s || R₁ || R₂

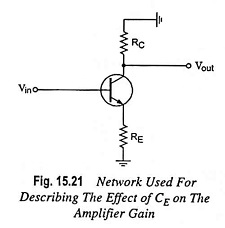
The effect of C_E on the gain is best described in a quantitative manner by recalling that the gain for the configuration shown in Fig. 15.21 is given as

The maximum gain will obviously be for R_E = 0 Ω. At low frequencies, with the bypass capacitor C_E is its “open-circuit” equivalent state, all of R_E appears in the above voltage gain equation, resulting in minimum gain.

With the increase in frequency, the reactance of capacitor C_E decreases, reduc­ing the parallel impedance of R_E and C_E until the resistor R_E is effectively “shorted out” by C_E. The result is a maximum or midband gain given by equation A_v = -R_C/r_e. At f_LE the gain will be 3 dB down the midband value determined with R_E “shorted out.”