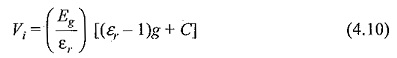Breakdown in Composite Dielectrics:
It is difficult to imagine a complete insulation system in an electrical equipment which does not consist of more than one type of insulation. If an insulation system as a whole is considered, it will be found that more than one insulating material is used. These different materials can be in parallel with each other, such as air or SF6 gas in parallel with solid insulation or in series with one another. Such insulation systems are called Breakdown in Composite Dielectrics.
Properties of Composite Dielectrics:
A Breakdown in Composite Dielectrics generally consists of a large number of layers arranged one over the other. This is called “the layered construction” and is widely used in cables, capacitors and transformers: Three properties of composite dielectrics which are important to their performance are given below.
Effect of Multiple Layers:
The simplest composite dielectric consists of two layers of the same material. Here, advantage is taken of the fact that two thin sheets have a higher dielectric strength than a single sheet of the same total thickness. The advantage is particularly significant in the case of materials having a wide variation in dielectric strength values measured at different points on its surface.
Effect of Layer Thickness:
Increase in layer thickness normally gives increased breakdown voltage. In a layered construction, breakdown channels occur at the interfaces only and not directly through another layer. Also, a discharge having penetrated one layer cannot enter the next layer until a part of the interface also attains the potential which can produce an electric field stress comparable to that of the discharge channel.
The use of layered construction is very important in the case of insulating paper since the paper thickness itself varies from point to point and consequently the dielectric strength across its surface is not homogeneous. The differences in the thickness impart a rough surface to the paper which can produce an electric field stress comparable to that of the discharge channel. The rough surface of the paper also helps in better impregnation when tightly wound. On the other hand, the existence of areas with lower thickness in the paper can cause breakdown at these points at considerably lower voltages.
Various investigations on Breakdown in Composite Dielectrics have shown that
- the discharge inception voltage depends on the thickness of the solid dielectric, as well as on the dielectric constant of both the liquid and solid dielectric, and
- the difference in the dielectric constants between the liquid and solid dielectrics does not significantly affect the rate of change of electric field at the electrode edge with the change in the dielectric thickness.
Effect of Interfaces:
The interface between two dielectric surfaces in a Breakdown in Composite Dielectrics system plays an important role in determining its pre-breakdown and breakdown strengths. Discharges usually occur at the interfaces and the magnitude of the discharge depends on the associated surface resistance and capacitance. When the surface conductivity increases, the discharge magnitude also increases, resulting in damage to the dielectric.
In a Breakdown in Composite Dielectrics, it is essential to maintain low dielectric losses because they normally operate at high electric stresses. However, even in an initially pure dielectric liquid, when used under industrial conditions for impregnating solid dielectrics, impurities arise, resulting in increased dielectric losses. The effect of various impurities in causing the breakdown of composite dielectrics is discussed in the next section.
Mechanisms of Breakdown in Composite Dielectrics:
As mentioned in the earlier section, if dielectric losses are low the cumulative heat produced will be low and thermal breakdown will not occur. However, several other factors can cause short and long time breakdown.
Short-term Breakdown:
If the electric field stresses are very high, failure may occur in seconds or even faster without any substantial damage to the insulating surface prior to breakdown. It has been observed that breakdown results from one or more discharges when the applied voltage is close to the observed breakdown value. There exists a critical stress in the volume of the dielectric at which discharges of a given magnitude can enter the insulation from the surface and propagate rapidly into its volume to cause breakdown. Experiments with single discharges on an insulating material have shown that breakdown occurs more rapidly when the electric field in the insulation is such that it assists the charged particles in the discharge to penetrate into the insulation, than when the field opposes their entry. Breakdown was observed to occur more readily when the bombarding particles are electrons, rather than positive ions. In addition, there are local field intensifications due to the presence of impurities and variations in the thickness of solid insulation and these local field intensifications play a very important role in causing breakdown under high field conditions; the actual effect being dependent on the field in the insulation before the discharge impinges on it. A more detailed description is given in the book by Bradley.
Long-term Breakdown:
Long-term breakdown is also called the ageing of insulation. The principal effects responsible for the ageing of the insulation which eventually leads to breakdown arise from the thermal processes and partial discharges. Partial discharges normally occur within the volume of the composite insulation systems. In addition, the charge accumulation and conduction on the surface of the insulation also contributes significantly towards the ageing and failure of insulation.
(i) Ageing and breakdown due to partial discharges:
During the manufacture of composite insulation, gas filled cavities will be present within the dielectric or adjacent to the interface between the conductor and the dielectric. When a voltage is applied to such a system, discharges occur within the gas-filled cavities. These discharges are called the “partial discharges” and involve the transfer of electric charge between the two points in sufficient quantity to cause the discharge of the local capacitance. At a given voltage, the impact of this charge on the dielectric surface produces a deterioration of the insulating properties, in many ways, depending on the geometry of the cavity and the nature of the dielectric. The study of breakdown by partial discharges is very important in industrial systems.
The degree of ageing depends on the discharge inception voltage, Vi and the discharge magnitude. It has been shown that Vi is strongly dependent on the permittivity of the dielectric εr and the thickness of the cavity g. Vi can be estimated approximately by
where Eg is the breakdown stress of the cavity air gap of thickness g and t is the thickness of the dielectric in series with the cavity. For any given arrangement (g+ t) will be constant. Let us call this constant as C. Then, the above equation can be written as
Differentiating, we get
Here, Eg is always positive and dE/dg is always negative or zero.
In obtaining the above expressions, two assumptions have been made. One is that in the cavity the stress Eg = εr . E, where E is the electric field across the dielectric. The other is that within the cavity Eg(max)/Eg = 1. When these assumptions are valid, the Paschen’s law can be used to explain the breakdown of the gas gap (cavity). However, the validity of the above assumptions depends upon the shape and dimensions of the cavity.
For the breakdown of the gas in the cavity to occur, the discharge has to start at one end and progress to the other end. As the discharge progresses, the voltage across the cavity drops due to charge accumulation on the cavity surface towards which it is progressing, and often the discharge gets extinguished. The discharge extinction voltage depends on the conditions inside the cavity. The Discharge causes a rise in the temperature and pressure of the gas in the cavity and gaseous deterioration products are also formed. At high frequencies, when the discharges occur very rapidly, these may cause the extinction voltage levels to reach lower values in spite of the erosion of the cavity walls.
From the above analysis the following conclusions can be arrived at
- for very small cavities, Vi decreases as the cavity depth increases, following the Paschen curve of gas breakdown.
- in spite of the erosion in the cavity walls, breakdown will not occur and the life of the insulation is very long if the applied voltage is less than 2 Vi.
- for applied voltages greater than 2 Vi, erosion is faster and therefore ageing of the insulation is quicker.
- the total capacitance of the cavity is not discharged as a single event but as a result of many discharges, each discharge involving only a small area of the cavity wall determined by the conductivity of the cavity surface in the region of the discharge.
Further details on ageing and breakdown due to partial discharges can be had from the book edited by A. Bradley (1984).
(ii) Ageing and breakdown due to accumulation of charges on insulator surfaces:
During discharges at the solid or liquid or solid-gas or solid-vacuum interfaces, certain quantity of charge (electrons or positive ions) gets deposited on the solid insulator surface. The charge thus deposited can stay there for very long duration, lasting for days or even weeks. The presence of this charge increases the surface conductivity thereby increasing the discharge magnitude in subsequent discharges. Increased discharge magnitude in subsequent discharges causes damage to the dielectric surface. Experiments using electro-photography and other methods have shown that transverse discharges occur on the faces of the dielectric, and these discharges cause a large area to be discharged instantaneously. Charges that exist in surface conductivity are due to the discharges themselves such that changes in discharge magnitude will occur spontaneously during the life of a dielectric.
It has been generally observed that the discharge characteristics change with the life of the insulation. This can be explained as follows: for clean surfaces, at the discharge inception voltage Vi, the discharge characteristic depends on the nature of the dielectric, its size and shape. The discharge normally consists of a large number of comparatively small discharges originating from sites on the insulator surface where the necessary discharge condition exists. After some time, erosion at these sites causes the discharges to decrease in number as well as in magnitude, and consequently total extinction may occur. With the passage of time, the phenomena involved become complex because the charges from the surface-induced conductivity add to the charge accumulation in the bulk due to partial discharges.