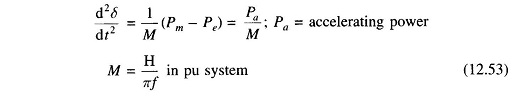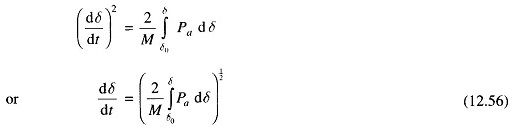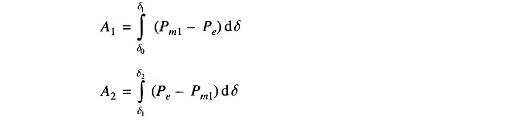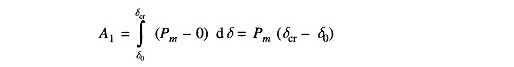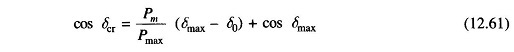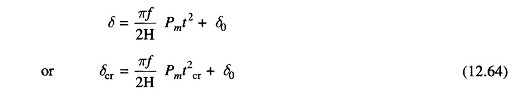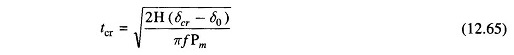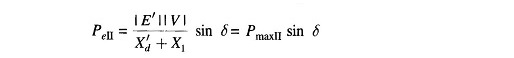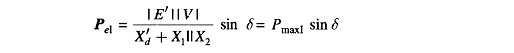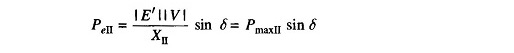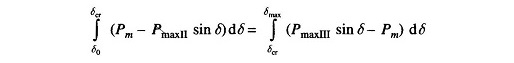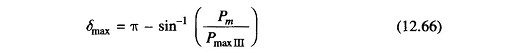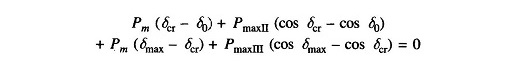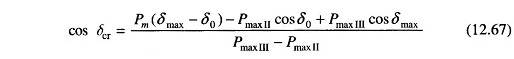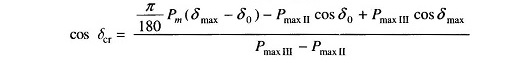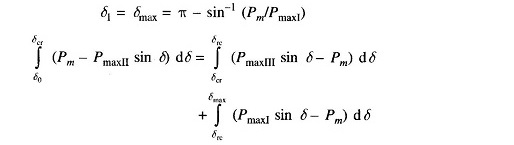Equal Area Criterion in Power System:

In a system where one machine is swinging with respect to an infinite bus, it is possible to study transient stability by means of a simple Equal Area Criterion in Power System, without resorting to the numerical solution of a swing equation.

Consider the swing equation

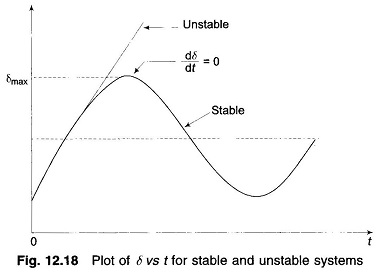
If the system is unstable δ continues to increase indefinitely with time and the machine loses synchronism. On the other hand, if the system is stable, δ(t) performs oscillations (nonsinusoidal) whose amplitude decreases in actual practice because of damping terms (not included in the swing equation). These two situations are shown in Fig. 12.18. Since the system is non-linear, the nature of its response [δ(t)] is not unique and it may exhibit instability in a fashion different from that indicated in Fig. 12.18, depending upon the nature and severity of disturbance. However, experience indicates that the response δ(t) in a power system generally falls in the two broad categories as shown in the figure. It can easily be visualized now (this has also been stated earlier) that for a stable system, indication of stability will be given by observation of the first swing where δ will go to a maximum and will start to reduce. This fact can be stated as a stability criterion, that the system is stable if at some time

and is unstable, if

for a sufficiently long time (more than 1 s will generally do).

The stability criterion for power systems stated above can be converted into a simple and easily applicable form for a single machine infinite bus system.

Multiplying both sides of the swing equation by (2 dδ/dt),  we get

Integrating, we have

where δ₀ is the initial rotor angle before it begins to swing due to disturbance.

From Eqs. (12.55) and (12.56), the condition for stability can be written as

or

The condition of stability can therefore be stated as: the system is stable if the area under P_a (accelerating power) – δ curve reduces to zero at some value of δ. In other words, the positive (accelerating) area under P_a — δ curve must equal the negative (decelerating) area and hence the name ‘equal area’ criterion of stability.

To illustrate the Equal Area Criterion in Power System of stability, we now consider several types of disturbances that may occur in a single machine infinite bus bar system.

Sudden Change in Mechanical Input:

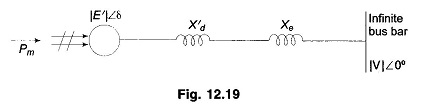
Figure 12.19 shows the transient model of a single machine tied to infinite bus bar. The electrical power transmitted is given by

Under steady operating condition

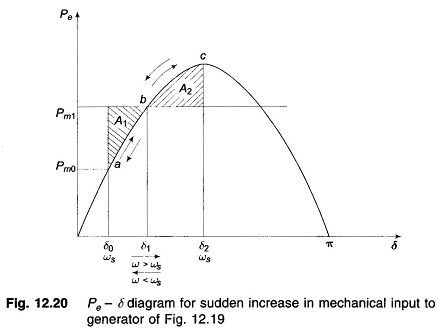
This is indicated by the point a in the P_e – δ diagram of Fig. 12.20.

Let the mechanical input to the rotor be suddenly increased to P_m1 (by opening the steam valve). The accelerating power P_a = P_m1 – P_e causes the rotor speed to increase (ω > ω_s) and so does the rotor angle. At angle δ₁, P_a = P_m1 – P_e (=P_max sin δ₁) = 0 (state point at b) but the rotor angle continues to increase as ω > ω_s. P_a now becomes negative (decelerating), the rotor speed begins to reduce but the angle continues to increase till at angle δ_2, ω = ω_s once again (state point at c. At c), the decelerating area A₂ equals the accelerating area A₁ (areas are shaded), i.e.,

Since the rotor is decelerating, the speed reduces below ω_s and the rotor angle begins to reduce. The state point now traverses the P_e – δ curve in the opposite direction as indicated by arrows in Fig. 12.20. It is easily seen that the system oscillates about the new steady state point b (δ = δ₁) with angle excursion up to δ₀ and δ₂ on the two sides. These oscillations are similar to the simple harmonic motion of an inertia-spring system except that these are not sinusoidal.

As the oscillations decay out because of inherent system damping (not modelled), the system settles to the new steady state where

From Fig. 12.20, areas A₁ and A₂ are given by

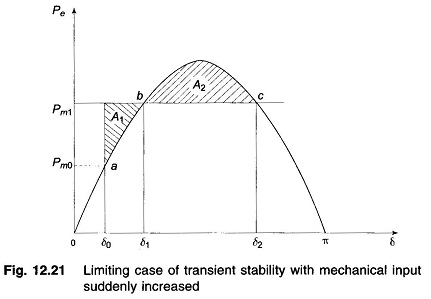
For the system to be stable, it should be possible to find angle δ₂ such that A₁ = A₂. As P_m1 is increased, a limiting condition is finally reached when A₁ equals the area above the P_m1 line as shown in Fig. 12.21. Under this condition, δ₂ acquires the maximum value such that

Any further increase in P_m1 means that the area available for A₂ is less than A₁, so that the excess kinetic energy causes δ to increase beyond point c and the decelerating power changes over to accelerating power, with the system consequently becoming unstable. It has thus been shown by use of the Equal Area Criterion in Power System that there is an upper limit to sudden increase in mechanical input (P_m1 – P_m0) for the system in question to remain stable.

It may also be noted from Fig. 12.21 that the system will remain stable even though the rotor may oscillate beyond δ = 90°, so long as the Equal Area Criterion in Power System is met. The condition of δ = 90° is meant for use in steady state stability only and does not apply to the transient stability case.

Effect of Clearing Time on Stability:

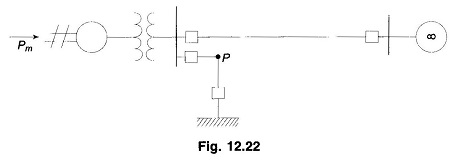
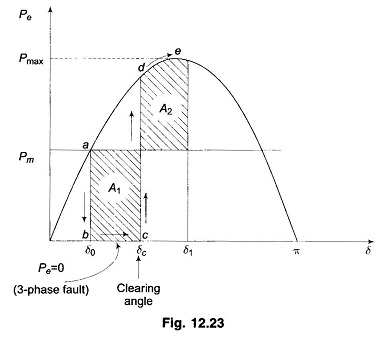
Let the system of Fig. 12.22 be operating with mechanical input P_m at a steady angle of δ₀ (P_m = P_e) as shown by the point a on the P_e – δ diagram of Fig. 12.23. If a 3-phase fault occurs at the point P of the outgoing radial line, the electrical output of the generator instantly reduces to zero, i.e., P_e = 0 and the state point drops to b. The acceleration area A₁ begins to increase and so does the rotor angle while the state point moves along bc. At time t_c corresponding to angle δ_c the faulted line is cleared by the opening of the line circuit breaker. The values of t_c and δ_c are respectively known as clearing time and clearing angle. The system once again becomes healthy and transmits P_e = P_max sin δ i.e. the state point shifts to d on the original P_e – δ curve. The rotor now decelerates and the decelerating area A₂ begins while the state point moves along de.

If an angle δ₁ can be found such that A₂ = A₁, the system is found to be stable. The system finally settles down to the steady operating point a in an oscillatory manner because of inherent damping.

The value of clearing time corresponding to a clearing angle can be established only by numerical integration except in this simple case. The Equal Area Criterion in Power System therefore gives only qualitative answer to system stability as the time when the breaker should be opened is hard to establish.

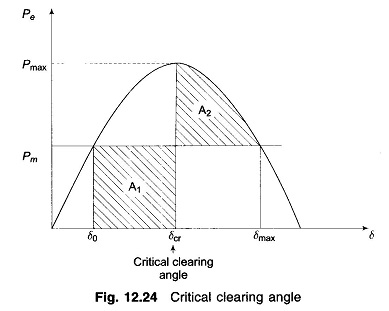
As the clearing of the faulty line is delayed, A₁ increases and so does δ₁ to find A₂ = A₁ till δ₁ = δ_max as shown in Fig. 12.24. For a clearing time (or angle) larger than this value, the system would be unstable as A₂ < A_1. The maximum allowable value of the clearing time and angle for the system to remain stable are known respectively as critical clearing time and angle.

For this simple case (P_e = 0 during fault), explicit relationships for δ_c (critical) and t_c (critical) are established below. All angles are in radians.

It is easily seen from Fig. 12.24 that

and

Now

and

For the system to be stable, A₂ = A₁, which yields

where

• δ_cr = critical clearing angle

Substituting Eqs. (12.59) and (12.60) in Eq. (12.61), we get

During the period the fault is persisting, the swing equation is

Integrating twice

where

• t_cr = critical clearing time
• δ_cr = critical clearing angle

From Eq. (12.64)

where δ_cr is given by the expression of Eq. (12.62)

An explicit relationship for determining t_cr is possible in this case as during the faulted condition P_e = 0 and so the swing equation can be integrated in closed form. This will not be the case in most other situations.

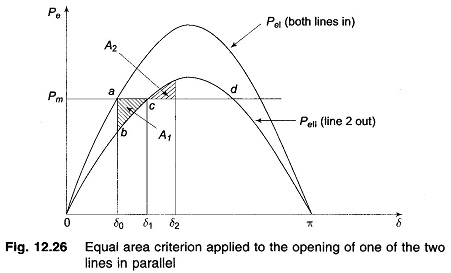
Sudden Loss of One of Parallel Lines:

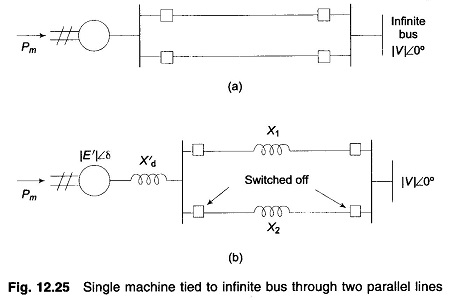
Consider now a single machine tied to infinite bus through two parallel lines as in Fig. 12.25a. Circuit model of the system is given in Fig. 12.25b.

Let us study the transient stability of the system when one of the lines is suddenly switched off with the system operating at a steady load. Before switching off, power angle curve is given by

Immediately on switching off line 2, power angle curve is given by

Both these curves are plotted in Fig. 12.26, wherein P_maxII < P_maxI as (X′_d+X₁) > (X′_d+X₁ II X₂). The system is operating initially with a steady power transfer P_e = P_m at a torque angle δ₀ on curve I.

Immediately on switching off line 2, the electrical operating point shifts to curve II (point b). Accelerating energy corresponding to area A₁ is put into rotor followed by decelerating energy for δ > δ₁. Assuming that an area A₂ corresponding to decelerating energy (energy out of rotor) can be found such that A₁ = A₂, the system will be stable and will finally operate at c corresponding to a new rotor angle δ₁ > δ₀. This is so because a single line offers larger reactance and larger rotor angle is needed to transfer the same steady power.

It is also easy to see that if the steady load is increased (line P_m is shifted upwards in Fig. 12.26), a limit is finally reached beyond which decelerating area equal to A₁ cannot be found and therefore, the system behaves as an unstable one. For the limiting case of stability, δ₁ has a maximum value given by

which is the same condition as in the previous example.

Sudden Short Circuit on One of Parallel Lines:

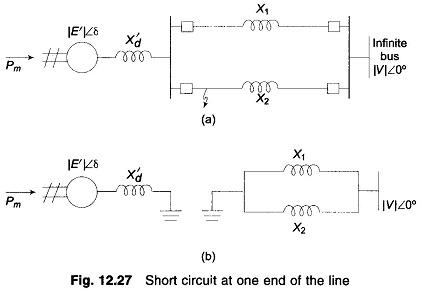
Case a: Short circuit at one end of line

Let us now assume the disturbance to be a short circuit at the generator end of line 2 of a double circuit line as shown in Fig. 12.27a. We shall assume the fault to be a three-phase one.

Before the occurrence of a fault, the power angle curve is given by

which is plotted in Fig. 12.25.

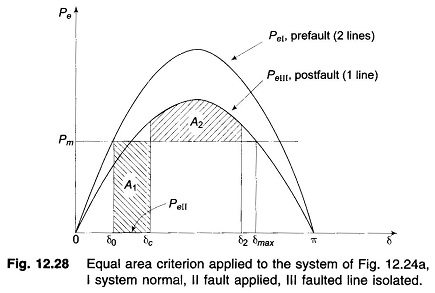
Upon occurrence of a three-phase fault at the generator end of line 2 (see Fig. 12.24a), the generator gets isolated from the power system for purposes of power flow as shown by Fig. 12.27b. Thus during the period the fault lasts,

The rotor therefore accelerates and angles δ increases. Synchronism will be lost unless the fault is cleared in time.

The circuit breakers at the two ends of the faulted line open at time t_c (corresponding to angle δ_c), the clearing time, disconnecting the faulted line.

The power flow is now restored via the healthy line (through higher line reactance X₂ in place of X₁ || X₂), with power angle curve

Obviously, P_max|| < P_max|.  The rotor now starts to decelerate as shown in Fig. 12.28. The system will be stable if a decelerating area A₂ can be found equal to accelerating area A₁ before δ reaches the maximum allowable value δ_max. As area A₁ depends upon clearing time t_c (corresponding to clearing angle δ_c), clearing time must be less than a certain value (critical clearing time) for the system to be stable. It is to be observed that the Equal Area Criterion in Power System helps to determine critical clearing angle and not critical clearing time. Critical clearing time can be obtained by numerical solution of the swing equation.

It also easily follows that larger initial loading (P_m) increases A₁ for a given clearing angle (and time) and therefore quicker fault clearing would be needed to maintain stable operation.

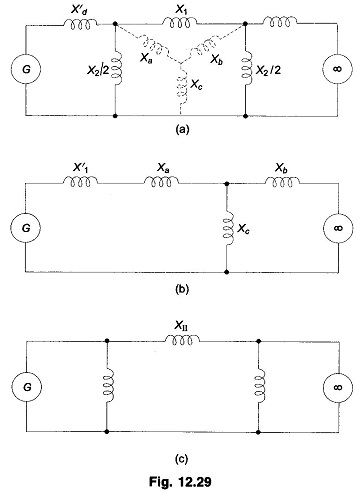
Case b: Short circuit away from line ends

When the fault occurs away from line ends (say in the middle of a line), there is some power flow during the fault though considerably reduced, as different from case a where P_eII = 0. Circuit model of the system during fault is now shown in Fig. 12.29a. This circuit reduces to that of Fig. 12.29c through one delta-star and one star-delta conversion. The power angle curve during fault is therefore given by

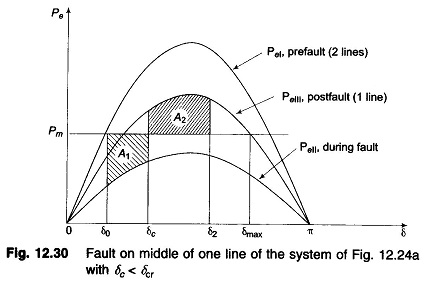
P_eI and P_eIII as in Fig. 12.28 and P_eII as obtained above are all plotted in Fig. 12.30. Accelerating area A₁ corresponding to a given clearing angle δ_c is less

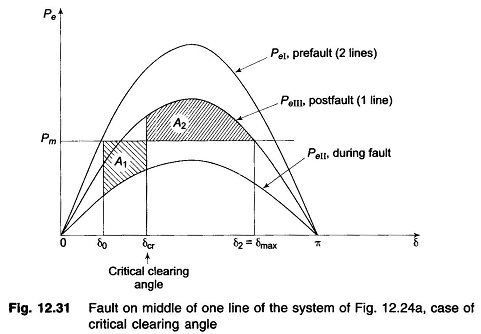
in this case, than in case a, giving a better chance for stable operation. Stable system operation is shown in Fig. 12.30, wherein it is possible to find an area A₂ equal to A₁ for δ₂ < δ_max. As the clearing angle δ_c is increased, area A₁ increases and to find A₂ = A₁, δ₂ increases till it has a value δ_max, the maximum allowable for stability. This case of critical clearing angle is shown in Fig. 12.31.

Applying Equal Area Criterion in Power System to the case of critical clearing angle of Fig. 12.31,

we can write

where

Integrating, we get

or

or

Critical clearing angle can be calculated from Eq. (12.67) above. The angles in this equation are in radians. The equation modifies as below if the angles are in degrees.

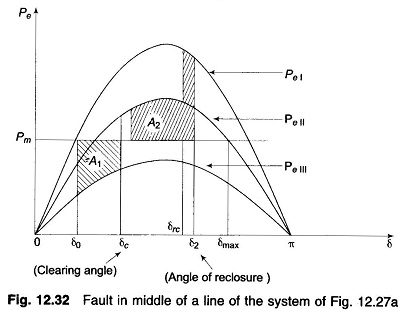
Case c: Reclosure

If the circuit breakers of line 2 are reclosed successfully (i.e., the fault was a transient one and therefore vanished on clearing the faulty line), the power transfer once again becomes

Since reclosure restores power transfer, the chances of stable operation improve. A case of stable operation is indicated by Fig. 12.32.

For critical clearing angle

where t_rc = t_cr + τ; τ = time between clearing and reclosure.