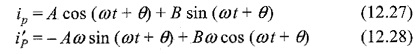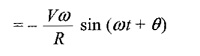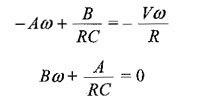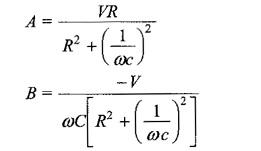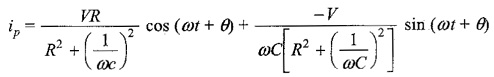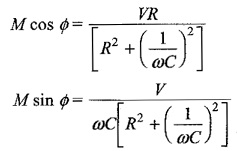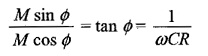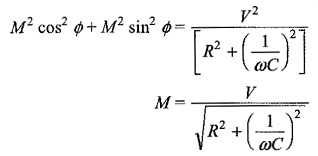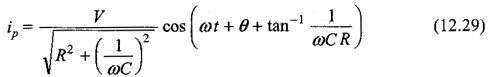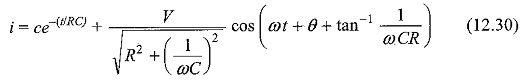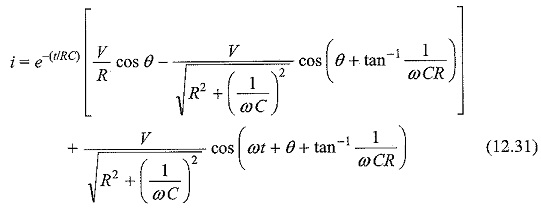Sinusoidal Response of RC Circuit:
Consider a Sinusoidal Response of RC Circuit consisting of resistance and capacitance in series as shown in Fig. 12.18.
The, switch, S, is closed at t = 0. At t = 0, a sinusoidal voltage V cos (ωt + θ) is applied to the RC Circuit, where V is the amplitude of the wave and θ is the phase angle. Applying Kirchhoff’s voltage law to the circuit results in the following differential equation.
The complementary function
The particular solution can be obtained by using undetermined coefficients.
Substituting Eqs 12.27 and 12.28 in Eq. 12.25, we get
Comparing both sides,
From which,
Substituting the values of A and B in Eq. 12.27, we have
Putting
To find M and Φ, we divide one equation by the other,
Squaring both equations and adding, we get
The particular current becomes
The complete solution for the current i = ic + ip
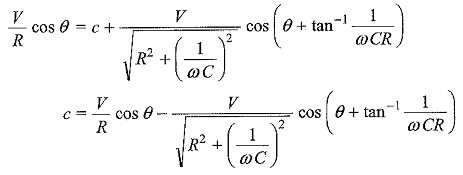
Since the capacitor does not allow sudden changes in voltages at t = 0, i = V/R cos θ
The complete solution for the current is