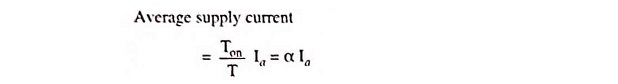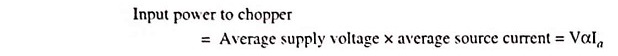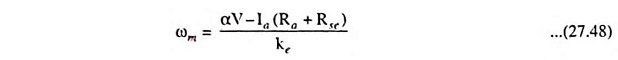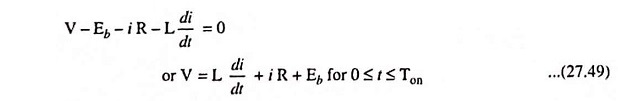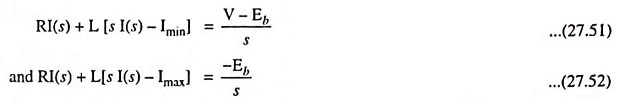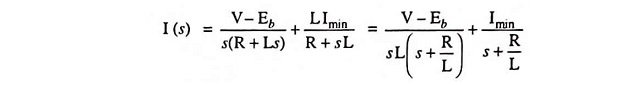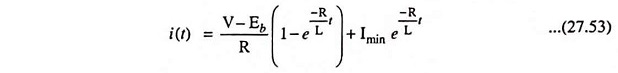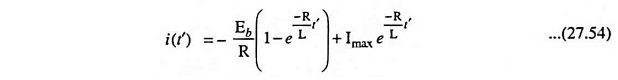Step Down Chopper with RLE Load Working Principle:

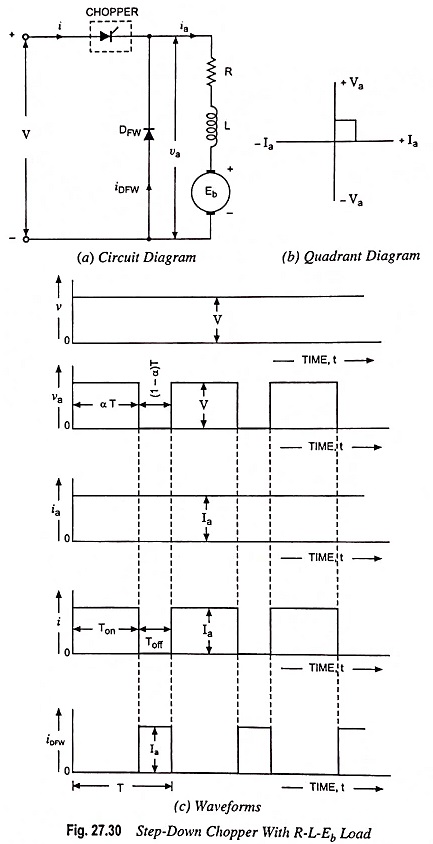
The choppers are widely employed for speed control of dc motors in industrial and traction drives. Figure 27.30 (a) shows the basic Step Down Chopper with RLE Load for the control of a dc series motor. The Step Down Chopper with RLE Load is shown to consist of a force-commutated thyristor, it could well be a transistor switch. It offers one-quadrant drive [Fig. 27.30 (b)]. Armature current is assumed continuous and ripple free. The waveforms for the source voltage V, armature terminal voltage v_a, armature current i_a, supply current i and freewheeling-diode current i_DFW are shown in Fig. 27.30 (c). From these waveforms we have

Average value of voltage across motor,

where α = Duty cycle = T_on/T and f = Chopping frequency = 1/T

For the motor armature circuit,

or Motor speed,

From above Eq. (27.48) it is obvious that the armature terminal voltage and therefore, speed of the dc motor can be controlled by varying the duty cycle α of the chopper.

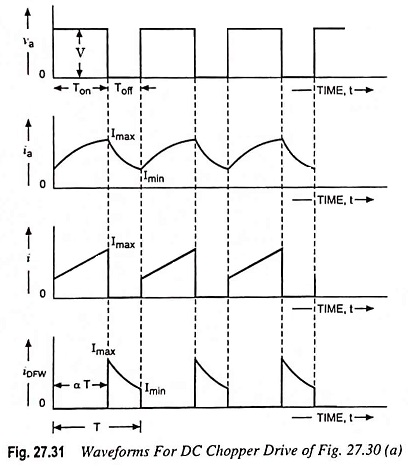
So far, armature current i_a has been assumed ripple free and accordingly waveforms in Fig. 27.30 (c) are drawn. But in practice, the motor armature current increases during chopper on period and decreases during chopper off period, as illustrated in Fig. 27.31.

For R-L-E_b type of load, E_b is load voltage (back emf of motor). When the chopper is switched on for a period T_on and power is supplied to R-L-E_b load. By using Kirchhoff’s voltage law (KVL) in the loop consisting of V, chopper and R-L-E_b load, we have

When chopper is off, the load current continues flowing through the freewheeling diode due to energy stored in the inductor. The KVL for the loop containing R-L-E_b and D_FW yields

The above Eqs. (27.49) and (27.50) can be solved by using Laplace transform. It is seen from Fig. 27.31 that initial value of load current is I_min for Eq. (27.49) and I_max for Eq. (27.50).

So Laplace transform of Eqs. (27.49) and (27.50) we have

From Eq. (27.51), we have

Laplace inverse of above equation is

Similarly the time-domain expression for current from Eq. (27.52) is

Here

• R = Armature resistance R_a + series field resistance R_se
• L = Armature inductance + series field inductance L_se.

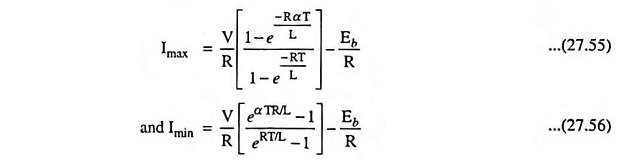
The values of maximum current I_max and minimum current I_min can be determined by using the fact that i (t) = I_min when t = 0 and i (t’) = I_max when t’ = 0. The values are found to be